Our AP Physics 1, Unit 6 test is based on the concepts of kinematics and dynamics of simple harmonic motion. Also, the study of a simple pendulum and spring-mass system is needed for this practice test. These topics are practical applications of Newton’s law of motion and kinematics.
Congratulations - you have completed .
You scored %%SCORE%% out of %%TOTAL%%.
Your performance has been rated as %%RATING%%
Your answers are highlighted below.
Question 1 |
A ball is in equilibrium inside a frictionless well, as shown below.
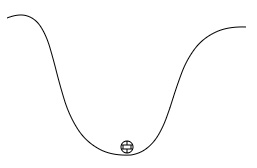
When the ball is displaced from its initial position, what will be the type of motion?
Oscillatory Motion but not necessarily Simple Harmonic Motion | |
Simple Harmonic Motion | |
Oscillatory Motion whose amplitude of motion decays in time | |
Depends upon the composition of the ball |
Question 1 Explanation:
When displaced the restoring force on the ball would be,
$f = mg\ \sinθ\ $(where $θ$ is the angle made by the normal with the vertical).
For general motion, $\sinθ$ is not $θ$ thus the motion is oscillatory but not necessarily simple harmonic.
$f = mg\ \sinθ\ $(where $θ$ is the angle made by the normal with the vertical).
For general motion, $\sinθ$ is not $θ$ thus the motion is oscillatory but not necessarily simple harmonic.
Question 2 |
Which of the below lengths of pendulums will have a period of $1.4\ s$?
(Use $g = 10\ m/s^2$)
$0.9\ m$ | |
$1.1\ m$ | |
$1.4\ m$ | |
$0.5\ m$ |
Question 2 Explanation:
$T = 2π \sqrt{\dfrac{l}{g}}$
Inverting the relation gives,
$l = \dfrac{gT^2}{4π^2}$
[Using $g = 10\ m/s^2$ and $T = 1.4\ s$]
$l = \dfrac{10×1.4^2}{4×π^2} = 0.49\ m$
Which is closest to Option D.
Inverting the relation gives,
$l = \dfrac{gT^2}{4π^2}$
[Using $g = 10\ m/s^2$ and $T = 1.4\ s$]
$l = \dfrac{10×1.4^2}{4×π^2} = 0.49\ m$
Which is closest to Option D.
Question 3 |
A spring is stretched by $1\ cm$ using a block of mass $2\ kg$ to make a simple harmonic oscillator. The spring constant is $32\ N/m$. Which of the below graphs correctly shows the displacement of the block?
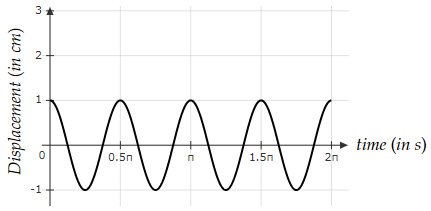 | |
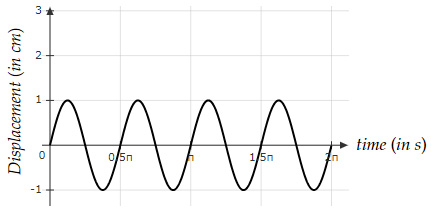 | |
 | |
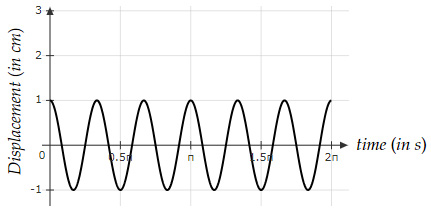 |
Question 3 Explanation:
$T = 2π \sqrt{\dfrac{m}{k}}$
[Using $m = 2\ kg$ and $k = 32\ N/m$]
$T = 2π \sqrt{\dfrac{2}{32}} = \dfrac{π}{2} s$
Since, $x = A \cos \dfrac{2πt}{T}$
$→ x ∝\cos4t$
Which has a period of $\dfrac{π}{2}$ (Option A).
[Using $m = 2\ kg$ and $k = 32\ N/m$]
$T = 2π \sqrt{\dfrac{2}{32}} = \dfrac{π}{2} s$
Since, $x = A \cos \dfrac{2πt}{T}$
$→ x ∝\cos4t$
Which has a period of $\dfrac{π}{2}$ (Option A).
Question 4 |
A mass of $3.5\ kg$ is attached to a spring of constant $30\ N/m$. The spring is then pushed from its equilibrium position by $3.5\ cm$. What will be the velocity of the mass when it is $1\ cm$ away from its equilibrium position?
$8.25\ cm/s$ | |
$11.2\ cm/s$ | |
$9.81\ cm/s$ | |
$12.5\ cm/s$ |
Question 4 Explanation:
$v = \sqrt{\dfrac{k}{m} (A^2-x^2 )}$
[Using $k = 30 N/m,\ m = 3.5 kg, $ $ A = 3.5 \ cm$ and $x = 1 \ cm$]
$v = \sqrt{\dfrac{30}{3.5} (3.5^2-1^2 )}$
$v = 9.81\ cm/s$
[Using $k = 30 N/m,\ m = 3.5 kg, $ $ A = 3.5 \ cm$ and $x = 1 \ cm$]
$v = \sqrt{\dfrac{30}{3.5} (3.5^2-1^2 )}$
$v = 9.81\ cm/s$
Question 5 |
Luis draws the graph of maximum potential energy $PE_{max}$ as a function of spring constant $k$ for a spring-mass harmonic oscillator.
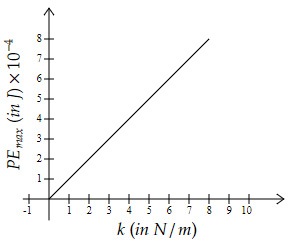
Which of the below quantities are correctly calculated from the graph?
Choose two correct answers:
The maximum amplitude of the oscillator is $\sqrt{2} \ cm$ | |
The value of $PE_{max}$ for any k is of the form $2k\ J$ | |
The kinetic energy of the mass is equal to $(10^{-4}-0.5x^2 )k\ J$ for any general $k$ and $x$ in m | |
The maximum velocity of the mass for $k = 2\ N/m$ is $3.4\ cm/s$ |
Question 5 Explanation:
$\dfrac{PE_{max}}{k} = 10^{-4} → \dfrac{A^2}{2} $ $ = \dfrac{1}{10^4}$
$A = \sqrt{2} \ cm$
Option A → Correct
Option B → Incorrect (Answer should be $10^{-4} k\ J$)
Kinetic energy $= \dfrac{1}{2} kA^2-\dfrac{1}{2} kx^2$
[Using $\dfrac{A^2}{2} = 10^{-4}$]
Kinetic energy $= (10^{-4}-0.5x^2 )k\ J$
Option C → Correct
Option D → Incorrect (Velocity cannot be calculated using the graph unless mass is known)
$A = \sqrt{2} \ cm$
Option A → Correct
Option B → Incorrect (Answer should be $10^{-4} k\ J$)
Kinetic energy $= \dfrac{1}{2} kA^2-\dfrac{1}{2} kx^2$
[Using $\dfrac{A^2}{2} = 10^{-4}$]
Kinetic energy $= (10^{-4}-0.5x^2 )k\ J$
Option C → Correct
Option D → Incorrect (Velocity cannot be calculated using the graph unless mass is known)
Question 6 |
A simple pendulum is used to measure the gravitational field strength on the surface of another planet. The time period of a pendulum of length $1\ m$ is $2.8\ s$ on the planet. By what factor is the gravitational field lesser on the planet as compared to Earth?
2 | |
3 | |
4 | |
5 |
Question 6 Explanation:
$T = 2π \sqrt{\dfrac{l}{g}}$
Inverting the relation,
$g = \dfrac{4π^2 l}{T^2}$
[Using $l = 1 \ m$ and $T = 2.8\ s$]
$g = \dfrac{(4π^2×1)}{2.8^2}$
$g = 5.03\ m/s^2$
Comparing with the $g$ of Earth,
Ratio $= 10 : 5.03 \; ≈ \; 2:1$
Inverting the relation,
$g = \dfrac{4π^2 l}{T^2}$
[Using $l = 1 \ m$ and $T = 2.8\ s$]
$g = \dfrac{(4π^2×1)}{2.8^2}$
$g = 5.03\ m/s^2$
Comparing with the $g$ of Earth,
Ratio $= 10 : 5.03 \; ≈ \; 2:1$
Question 7 |
Tyler sets up the below apparatus to find the spring constant $k$ of the spring.
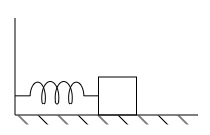
Also, he uses a stopwatch and a weighing scale to measure the time period of one oscillation and the mass of each block attached to the spring. He performs the experiment at least 5 times with each available block of mass $m$ and calculates $k$ for each $m$ and $T$.
Which of the following most likely represents Tyler’s observation?
The value of $k$ is exactly the same for all experiments | |
The value of $k$ varies slightly with each experiment | |
The value of $k$ changes only when either $m$ or $T$ is changed in the experiment | |
The value of $k$ varies appreciably for all the experiments |
Question 7 Explanation:
When Tyler measures $k$ in different experimental conditions he must obtain identical $k$ irrespective of the time period and the mass since the spring used is the same.
However, in all experiments some errors are always present so the value of $k$ must vary slightly each time.
Option B is correct.
However, in all experiments some errors are always present so the value of $k$ must vary slightly each time.
Option B is correct.
Question 8 |
Two waves (sine or cosine) are said to be in phase when they increase or decrease together at all times. When one wave increases and the other decreases at all times, they are said to be out of phase. Identify which of the below statements regarding Simple Harmonic Motion are correct.
The velocity and acceleration are in phase | |
The displacement and acceleration are in phase | |
The velocity and displacement are out of phase | |
The displacement and acceleration are out of phase |
Question 8 Explanation:
Displacement graph
Velocity graph
Acceleration graph
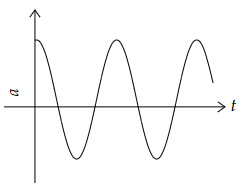
From the above graphs, note that $a$ and $x$ are out of phase.

Velocity graph

Acceleration graph

From the above graphs, note that $a$ and $x$ are out of phase.
Question 9 |
Which of the options below represents the magnitude of velocities in increasing order if the graph below shows the potential energy of the particle performing simple harmonic motion?
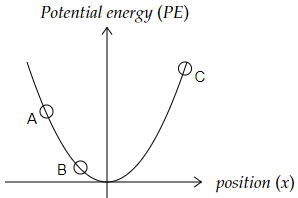
$v_c < v_b < v_a$ | |
$v_c < v_a < v_b$ | |
$v_b < v_a < v_c$ | |
$v_a < v_b < v_c$ |
Question 9 Explanation:
Kinetic energy of the particle
$= E-\dfrac{1}{2} kx^2$
Thus, more the potential energy lesser is the kinetic energy (and thus velocity):
$PE_c > PE_a > PE_b$
$KE_c < KE_a < KE_b$
Thus, $v_c < v_a < v_b $
$= E-\dfrac{1}{2} kx^2$
Thus, more the potential energy lesser is the kinetic energy (and thus velocity):
$PE_c > PE_a > PE_b$
$KE_c < KE_a < KE_b$
Thus, $v_c < v_a < v_b $
Question 10 |
A block attached to a vertical spring extends it by $6\ cm$. The mass of the block is $1\ kg$. If this system is then rotated into a horizontal plane, what is the frequency of oscillations?
$4\ per\ sec$ | |
$3\ per\ sec$ | |
$1\ per\ sec$ | |
$2\ per\ sec$ |
Question 10 Explanation:
When vertically oriented, the weight and the spring force balance each other:
$kx = mg$
$→ k = \dfrac{mg}{x}$
[Using $m = 1\ kg, x = 6×10^{-2}\ m$ and $g = 10\ m/s^2$ ]
$k = \dfrac{1×10}{6×10^{-2}}$
$k = 166\ N/m$
When rotated into a horizontal plane, the system starts performing simple harmonic oscillations with a frequency of,
$f = \dfrac{1}{2π} \sqrt{\dfrac{k}{m}}$
$f = \dfrac{1}{2π} \sqrt{\dfrac{166}{1}}$
$f = 2.05\ per\ second$
$kx = mg$
$→ k = \dfrac{mg}{x}$
[Using $m = 1\ kg, x = 6×10^{-2}\ m$ and $g = 10\ m/s^2$ ]
$k = \dfrac{1×10}{6×10^{-2}}$
$k = 166\ N/m$
When rotated into a horizontal plane, the system starts performing simple harmonic oscillations with a frequency of,
$f = \dfrac{1}{2π} \sqrt{\dfrac{k}{m}}$
$f = \dfrac{1}{2π} \sqrt{\dfrac{166}{1}}$
$f = 2.05\ per\ second$
Once you are finished, click the button below. Any items you have not completed will be marked incorrect.
There are 10 questions to complete.
|
List |
Next Practice Test:
Unit 7: Torque & Rotational Motion >>
AP Physics 1 Main Menu >>
