Our AP Physics 1, Unit 3 test is focused on the concepts of the universal law of gravitation. Further concepts, like acceleration due to gravity, potential energy, motion of satellites, and dynamics of circular motion, are included as a part of the assessment. These topics are an application of Newton’s laws of motion and related concepts.
Congratulations - you have completed .
You scored %%SCORE%% out of %%TOTAL%%.
Your performance has been rated as %%RATING%%
Your answers are highlighted below.
Question 1 |
When Henry rotates an object (with the help of a string) in a vertical circle of fixed radius $r$, why does the centripetal force change at each point of the motion?
Choose two correct answers:
The components of the weight (along the tangent and towards the center) keeps on changing | |
The apparent weight of the object changes | |
The motion of the string affects the centripetal force acting on the object | |
The velocity of the object changes at each point |
Question 1 Explanation:
The Free body diagram of the object at any general point of the circle is,
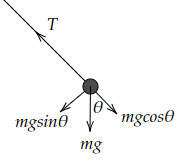
$T-mg \cosθ = \dfrac{mv^2}{r} $
Centripetal force is a function of the angle $θ$ (component of weight).
So, Option A is true.
And, Centripetal Force $= \dfrac{mv^2}{r}$
Thus, Centripetal Force changes whenever $v$ changes
So, Option D is true

$T-mg \cosθ = \dfrac{mv^2}{r} $
Centripetal force is a function of the angle $θ$ (component of weight).
So, Option A is true.
And, Centripetal Force $= \dfrac{mv^2}{r}$
Thus, Centripetal Force changes whenever $v$ changes
So, Option D is true
Question 2 |
A marble of mass $m_1$, tied to a string, performs uniform circular motion.
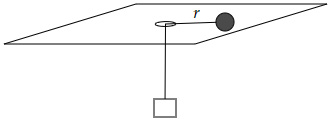
A block of mass $m_2$ is attached to the other end of the string. The velocity of the marble at which the tension force of the string is enough to balance the weight of the block is,
$\sqrt{2gr}$ | |
$\sqrt{\dfrac{2m_2 gr}{(m_1+m_2)}}$ | |
$\sqrt{\dfrac{m_1 gr}{m_2}}$ | |
$\sqrt{\dfrac{m_2 gr}{m_1}}$ |
Question 2 Explanation:
For Marble,
Tension $= T = \dfrac{m_1 v^2}{r}$
For Block,
Tension $= T = m_2 g$
Thus, $\dfrac{m_1 v^2}{r} = m_2 g → v^2 $ $ = \dfrac{m_2 gr}{m_1}$
Or $v = \sqrt{\dfrac{m_2 gr}{m_1}}$
Tension $= T = \dfrac{m_1 v^2}{r}$
For Block,
Tension $= T = m_2 g$
Thus, $\dfrac{m_1 v^2}{r} = m_2 g → v^2 $ $ = \dfrac{m_2 gr}{m_1}$
Or $v = \sqrt{\dfrac{m_2 gr}{m_1}}$
Question 3 |
Find the value of gravitational acceleration at a height of $2R_e$ from the Earth’s surface as a percentage of gravitational acceleration at the surface. (Here, $R_e$ is the radius of the Earth)
18.3% | |
14.2% | |
11.1% | |
9.9% |
Question 3 Explanation:
$g = \dfrac{GM}{R_e^2}$
While,
$g_h = \dfrac{GM}{(R_e+2R_e )^2} $ $ = \dfrac{GM}{9R_e^2}$
$\dfrac{g_h}{g} = \dfrac{GM}{9R_e^2}÷\dfrac{GM}{R_e^2} $ $ = \dfrac{1}{9}$
Thus, $\dfrac{g_h}{g} = 11.1 \% $
While,
$g_h = \dfrac{GM}{(R_e+2R_e )^2} $ $ = \dfrac{GM}{9R_e^2}$
$\dfrac{g_h}{g} = \dfrac{GM}{9R_e^2}÷\dfrac{GM}{R_e^2} $ $ = \dfrac{1}{9}$
Thus, $\dfrac{g_h}{g} = 11.1 \% $
Question 4 |
Andrew draws a schematic diagram for astronomical objects.
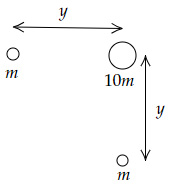
What is the net gravitational force acting on the heavier object?
$\dfrac{10\sqrt{2} Gm^2}{y^2}$ $\text{along the diagonal}$ | |
$\dfrac{10 Gm^2}{y^2}$ $\text{towards the left}$ | |
$\dfrac{10\sqrt{3} Gm^2}{y^2}$ $\text{along the diagonal}$ | |
$\dfrac{10 Gm^2}{y^2}$ $\text{coming out of the plane}$ |
Question 4 Explanation:
Force of gravity on $10\ m$ is equal to the vector sum of the forces from the left and bottom by $m$ and $m$
Force from left $F_l = \dfrac{10Gm^2}{y^2}$
Force from bottom $F_b = \dfrac{10Gm^2}{y^2}$
Net force = $\sqrt{F_l^2+F_b^2} = \dfrac{10\sqrt{2} Gm^2}{y^2}$
(along the diagonal)
Force from left $F_l = \dfrac{10Gm^2}{y^2}$
Force from bottom $F_b = \dfrac{10Gm^2}{y^2}$
Net force = $\sqrt{F_l^2+F_b^2} = \dfrac{10\sqrt{2} Gm^2}{y^2}$
(along the diagonal)
Question 5 |
What is the apparent weight of an object inside a satellite?
Same as the actual weight | |
2 times the actual weight | |
½ times the actual weight | |
0 times the actual weight |
Question 5 Explanation:
An object feels its own weight due to the normal force acting on it.
Since inside a satellite all the objects are in free fall towards the Earth (each is moving with the same acceleration).
Hence, there are no normal forces acting on an object in a satellite.
Thus, the apparent weight is 0.
Since inside a satellite all the objects are in free fall towards the Earth (each is moving with the same acceleration).
Hence, there are no normal forces acting on an object in a satellite.
Thus, the apparent weight is 0.
Question 6 |
Two identical objects are in circular motion about the center (shown with a ×) due to gravitational force.
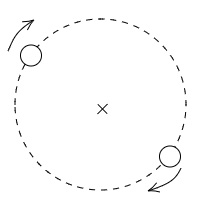
The mass of the two objects is $m$ and the radius of the circle is $a$. How many times (approximately) does the system appear in the same position as the initial state in a time interval $δt$?
$\dfrac{πa}{δt} \sqrt{\dfrac{a}{Gm}}$ | |
$\dfrac{2δt}{πa} \sqrt{\dfrac{Gm}{a}}$ | |
$\dfrac{δt}{2πa} \sqrt{\dfrac{Gm}{a}}$ | |
$\dfrac{3πa}{δt} \sqrt{\dfrac{a}{Gm}}$ |
Question 6 Explanation:
Gravitational Force = Centripetal Force
$\dfrac{Gm^2}{4a^2} = \dfrac{mv^2}{a}$
$v = \dfrac{1}{2} \sqrt{\dfrac{Gm}{a}}$
Time period $T = \dfrac{2πa}{v} $ $ = 2πa÷\dfrac{1}{2} \sqrt{\dfrac{Gm}{a}}$
$T = 4πa\sqrt{\dfrac{a}{Gm}}$
Thus, in time $δt$ the system is most likely to be in the same state
$\dfrac{δt}{(T/2)} = \dfrac{δt}{2πa} \sqrt{\dfrac{Gm}{a}}$ times.
$\dfrac{Gm^2}{4a^2} = \dfrac{mv^2}{a}$
$v = \dfrac{1}{2} \sqrt{\dfrac{Gm}{a}}$
Time period $T = \dfrac{2πa}{v} $ $ = 2πa÷\dfrac{1}{2} \sqrt{\dfrac{Gm}{a}}$
$T = 4πa\sqrt{\dfrac{a}{Gm}}$
Thus, in time $δt$ the system is most likely to be in the same state
$\dfrac{δt}{(T/2)} = \dfrac{δt}{2πa} \sqrt{\dfrac{Gm}{a}}$ times.
Question 7 |
A book weighs $28\ N$ on the surface of the Earth. What is the weight of the book on another planet whose radius is twice that of Earth and Mass thrice that of Earth?
$25\ N$ | |
$21\ N$ | |
$32\ N$ | |
$36\ N$ |
Question 7 Explanation:
Mass of the book $= \dfrac{28}{10} = 2.8\ kg$
(Using $g_e = 10\ m/s^2$)
Gravitational acceleration on the planet
$= \dfrac{GM_p}{R_p^2} = \dfrac{3GM_e}{4R_e^2} = 0.75\ g$
Weight on the planet
$= 0.75\ mg $ $ = 0.75×10×2.8 $ $ = 21\ N$
(Using $g_e = 10\ m/s^2$)
Gravitational acceleration on the planet
$= \dfrac{GM_p}{R_p^2} = \dfrac{3GM_e}{4R_e^2} = 0.75\ g$
Weight on the planet
$= 0.75\ mg $ $ = 0.75×10×2.8 $ $ = 21\ N$
Question 8 |
Which of the below graphs shows the correct relation between the distance and the magnitude of the gravitational potential energy of an object from the center of the planet?
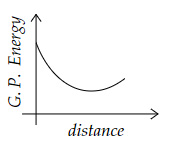 | |
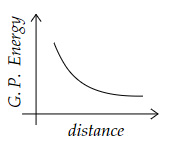 | |
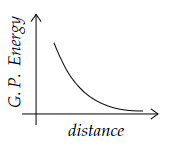 | |
 |
Question 8 Explanation:
$GPE = \dfrac{GMm}{r}$ for $ \, r ≥ R$ ($R$ is the radius of the planet)
(We are neglecting – sign as we need only the magnitude.)
Option A → Energy should decrease with increase in distance.
Option B → Energy should decrease to 0.
Option C → Correct plot.
Option D → Energy should decrease with increase in distance.
(We are neglecting – sign as we need only the magnitude.)
Option A → Energy should decrease with increase in distance.
Option B → Energy should decrease to 0.
Option C → Correct plot.
Option D → Energy should decrease with increase in distance.
Question 9 |
Questions 9 and 10 are based on the below information.
A car is moving along a curved road of radius R which has a banking angle $α$. The car is traveling at a speed of $u$. The coefficient of friction between the car and the road is $μ_k$.
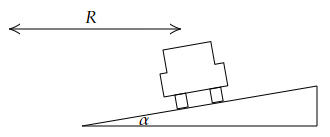
What should be the value of tanα if the force of friction between the car and the road is $0\ N$?
$\dfrac{u^2}{Rg}$ | |
$\dfrac{Rg}{u^2}$ | |
$\dfrac{u^2}{3Rg}$ | |
$\dfrac{4u^2}{5μ_k Rg}$ |
Question 9 Explanation:
The free body diagram for the car is,

Sum of vertical forces
$= mg-N \cosα $ $ = 0 → mg $ $ = N \cos\ α$
Sum of horizontal forces
$= N \sin\ α = \dfrac{mu^2}{R}$
Thus, $\tan\ α = \dfrac{u^2}{Rg}$

Sum of vertical forces
$= mg-N \cosα $ $ = 0 → mg $ $ = N \cos\ α$
Sum of horizontal forces
$= N \sin\ α = \dfrac{mu^2}{R}$
Thus, $\tan\ α = \dfrac{u^2}{Rg}$
Question 10 |
A car is moving along a curved road of radius R which has a banking angle $α$. The car is traveling at a speed of $u$. The coefficient of friction between the car and the road is $μ_k$.

What is the lowest possible speed at which a car can travel on this road for some banking angle $α$?
$\sqrt{Rg (\dfrac{\tan α+ μ_k}{1 + μ_k \tan α})}$ | |
$\sqrt{Rg (\dfrac{\tan α- μ_k}{1 - μ_k \tan α})}$ | |
$\sqrt{Rg (\dfrac{\tan α+ μ_k}{1 - μ_k \tan α})}$ | |
$\sqrt{Rg (\dfrac{\tan α- μ_k}{1 + μ_k \tan α})}$ |
Question 10 Explanation:
For lowest possible speed,
The free body diagram would be as follows,
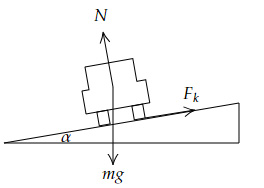
(Friction would prevent the car to slide inwards due to less speed)
Sum of vertical forces
$= N \cos α+F_k \sin α-mg = 0$
$= 0 → N \cos α+F_k \sin α $ $ = mg$
Sum of horizontal forces
$= N \sin α-F_k \cos α $ $ = \dfrac{mu^2}{R}$
On substituting
$F_k = μ_k N$ in the above two equations,
$N(\cos α+μ_k \sin α)$ $ = mg \ \text{ and } \ N(\sin α-μ_k \cos α ) $ $ = \dfrac{mu^2}{R}$
Solving for $u$,
$\dfrac {\sin α - μ_k \cos α}{\cos α + μ_k \sin α } = \dfrac{u^2}{Rg}$
Thus, $u_{min} $ $ = \sqrt{Rg (\dfrac{\tan α - μ_k}{1 + μ_k \tan α })} $
The free body diagram would be as follows,

(Friction would prevent the car to slide inwards due to less speed)
Sum of vertical forces
$= N \cos α+F_k \sin α-mg = 0$
$= 0 → N \cos α+F_k \sin α $ $ = mg$
Sum of horizontal forces
$= N \sin α-F_k \cos α $ $ = \dfrac{mu^2}{R}$
On substituting
$F_k = μ_k N$ in the above two equations,
$N(\cos α+μ_k \sin α)$ $ = mg \ \text{ and } \ N(\sin α-μ_k \cos α ) $ $ = \dfrac{mu^2}{R}$
Solving for $u$,
$\dfrac {\sin α - μ_k \cos α}{\cos α + μ_k \sin α } = \dfrac{u^2}{Rg}$
Thus, $u_{min} $ $ = \sqrt{Rg (\dfrac{\tan α - μ_k}{1 + μ_k \tan α })} $
Once you are finished, click the button below. Any items you have not completed will be marked incorrect.
There are 10 questions to complete.
|
List |
Next Practice Test:
Unit 4: Energy >>
AP Physics 1 Main Menu >>
