AP Calculus AB — Trig Integrals

The correct answer is (B). Since $\sin^3⁡(x)$ cannot be directly evaluated, we must break it into simpler functions as follows.

Use the trigonometric identity $\sin^2⁡(x) + \cos^2⁡(x) = 1$ to simplify the integral.

$\displaystyle\int(1 − \cos^2⁡(x))(\sin⁡(x))dx$

Expand the integrand.

$\displaystyle\int(\sin⁡(x)) − \cos^2⁡(x)\sin⁡(x))dx $

Using the additive property of integrals, we arrive at the form below.

$\displaystyle\int \sin⁡(x) dx~−$ $\displaystyle\int \cos^2⁡(x)\sin⁡(x)dx$

The first integral is equal to $−\cos⁡(x)$, but the second integral will require a $u$-substitution.

$u = \cos⁡(x)$

$du = −\sin⁡(x)dx$

$−\cos⁡(x) − [ − \displaystyle\int u^2 du]$

$−\cos⁡(x)+\dfrac{1}{3}\cos^3⁡(x) + C$

The correct answer is (A). Begin by using the power reduction identities for $\cos⁡(x)$ to rewrite $\sin^2⁡(x)$ and $\cos^2⁡(x)$.

$\displaystyle\int \left(\dfrac{1 − \cos(2x)}{2}\right)$ $\left(\dfrac{1 + \cos(2x)}{2}\right)dx$

Simplifying the expression above gives the form below.

$\dfrac{1}{4}\displaystyle\int − \cos^2(2x)dx$

Using the double angle identity again, the integral can be written in the form below.

$\dfrac{1}{4}\displaystyle\int \left[1 − \left(\dfrac{1 + \cos(4x)}{2}\right)\right]dx$

Using the properties of integrals, this expression can be simplified to the answer below.

$\dfrac{1}{4}\displaystyle\int \left[\dfrac{1}{2} − \dfrac{1}{2}\cos(4x)\right]dx$

$\dfrac{1}{4} \left[\dfrac{1}{2}x − \dfrac{1}{8}\sin(4x)\right] + C$

$\dfrac{1}{8}x − \dfrac{1}{32}\sin(4x) + C$

The correct answer is (D). Since the secant function is raised to an even power, the integrand can be rewritten in the form below.

$\displaystyle\int \sec^2(x)\tan^4(x)\sec^2(x)dx$

The appropriate $u$ expression for this integral is $\tan⁡(x)$.

$u = \tan(x)$

$du = \sec^2(x)dx$

Rewrite the integrand using a trigonometric identity.

$\displaystyle\int (\tan^2(x) + 1)$ $(\tan^4(x))\sec^2(x)dx$

Next, perform a $u$-substitution using $u = \tan⁡(x)$.

$\displaystyle\int (u^2 + 1)(u^4)du$

Expand and solve the integral.

$\displaystyle\int (u^6 + u^4)du$

$\dfrac{1}{7}u^7(x) + \dfrac{1}{5}u^5(x) + C$

Replace $u$ with $\tan⁡(x)$.

$\dfrac{1}{7}\tan^7(x) + \dfrac{1}{5}\tan^5(x) + C$

The correct answer is (B). Since the secant function is raised to the odd power, the integrand can be written in the form below.

$\displaystyle\int \sec^2(x) \tan^2(x) \sec(x) \tan(x) dx$

This can be rewritten using a $u$-substitution using $u = \sec⁡(x)$.

$u = \sec⁡(x)$

$du = \sec(x)\tan(x)dx$

Rewrite the integral in terms of $\sec⁡(x)$ using the trigonometric identity.


$\displaystyle\int [(\sec^2(x) − 1) (\sec^2(x)) \sec(x) \tan(x)] dx$


$\displaystyle\int [(\sec^4(x) − \sec^2(x)) \sec(x) \tan(x)] dx$

Perform the $u$-substitution.

$\displaystyle\int (u^4 − u^2)du$

Use the power rule of integrals to solve.

$\dfrac{1}{5}u^5 − \dfrac{1}{3}u^3 + C$

Replace $u$ with $\sec⁡(x)$.

$\dfrac{1}{5}\sec^5(x) − \dfrac{1}{3}\sec^3(x) + C$

The correct answer is (C).
Rewrite the expression in the form below.

$\displaystyle\int (\sec^2(x) − 1)(\tan(x))dx$

By simplifying, we arrive at the form below.

$\displaystyle\int (\sec^2(x) \tan(x) − \tan(x))dx$

Then using the $u$-substitution below, the first portion of the integral can be solved.

$u = \sec(x)$

$du = \sec(x)\tan(x)$

$\displaystyle\int u du − \displaystyle\int \tan(x)dx$

Using the basic integral rules and the special integral for $\tan⁡(x)$, we arrive at the form below.

$\dfrac{1}{2}u^2(x) − (− \ln |\cos(x)|) + C$

Replace $u$ with $\sec⁡(x)$.

$\dfrac{1}{2}\sec^2(x) + \ln |\cos(x)| + C$

The correct answer is (C). The derivative of the upper bound of the integral is $3$. Applying the second fundamental theorem:

$3(3x) \cdot 3 = 27x$

The correct answer is (B). The derivative of the upper bound is $−\sin⁡(x)$. Applying the second fundamental theorem, the answer is:

$−\sin^2(\cos(x)) \cdot \sin(x)$

The correct answer is (D). The derivative of the upper bound is $2x$. Applying the second fundamental theorem:

$\ln((x^2)^2 + x^2) \cdot 2x$

$= 2x \cdot \ln(x^4 + x^2)$

The correct answer is (A). The derivative of the upper bound is, using the power rule for derivatives, $\frac{−1}{x^2}$. Applying the second fundamental theorem:

$−\dfrac{1}{x^2}\left(\dfrac{3}{x} + 5 \right)$

The correct answer is (D). The derivative of the upper bound is $7x^6$. Applying the definition of the second fundamental theorem, the final answer is:

$7x^6\csc^2(3x^7)$

The correct answer is (A). Start by setting the equation equal and finding the intersections.

$x^2 + 3x + 2 = x + 5$

$x^2 + 2x − 3 = 0$

$(x + 3)(x − 1) = 0$

$x = −3, 1$

So, the bounds of integration are $−3$ and $1$. Note that $x + 5$ is above $x^2 + 3x + 2$ on the interval $[−3, 1]$ (from a graphing calculator). Therefore, the integral to find the bounded area is:

$\displaystyle\int_{−3}^1 [(x + 5) − (x^2 + 3x + 2)]dx$

$\displaystyle\int_{−3}^1 (−x^2 − 2x + 3)dx$

$−\dfrac{1}{3}x^3 − x^2 + 3x$

Applying the first fundamental theorem:

$\left[−\dfrac{1}{3}(1)^3 − (1)^2 + 3(1)\right]~−$ $\left[−\dfrac{1}{3}(−3)^3 − (−3)^2 + 3(−3)\right]$

$\left[−\dfrac{1}{3} − 1 + 3 \right] − [9 − 9 − 9]$ $= \dfrac{5}{3} + \dfrac{27}{3} = \dfrac{32}{3}$

The correct answer is (D). Sketching a graph of the two functions shows that $x^2 + 3x$ is above $\cos⁡(x)$ on the given interval.



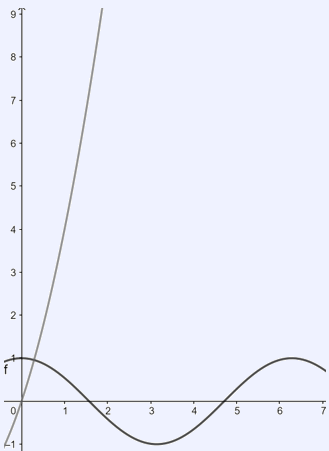
Thus, the integral to be evaluated is:

$\displaystyle\int_π^{2π} [(x^2 + 3x) − \cos(x)]dx$

$\frac{1}{3}x^3 + \frac{3}{2}x^2 − \sin(x)$

Applying the first fundamental theorem:

$\left[-\frac{1}{3}(2π)^3 + \frac{3}{2}(2π)^2 - \sin(2π)\right] − \left[-\frac{1}{3}(π)^3 + \frac{3}{2}(π)^2 − \sin(π)\right]$

$\frac{8}{3}π^3 + \frac{12}{2}π^2 − \left(\frac{1}{3}π^3 + \frac{3}{2}π^2 \right)$ $= \frac{7}{3}π^3 + \frac{9}{2}π^2$

The correct answer is (C). On this interval, $x^3$ is greater than $x^2$. Therefore, the area between the two curves can be calculated using the first fundamental theorem of calculus.

$A = \displaystyle\int_1^3 (x^3 − x^2) dx$

$\left[\dfrac{1}{4}x^4 − \dfrac{1}{3}x^3 \right]_1^3$

$A = \left[\dfrac{1}{4}(81) − \dfrac{1}{3}(27) \right] − \left[\dfrac{1}{4} − \dfrac{1}{3} \right]$ $= \dfrac{34}{3}$

The correct answer is (C). In order to find the intersection points, the two functions can be equated as follows.

$y = y^2 − 3 + 1$

$y = y^2 − 2$

$y = y^2 − y − 2 = 0$

$(y + 1)(y − 2) = 0$

$y = −1$, $y = 2$

The values found above indicate the bounds for the area enclosed on the $y$-axis. Thus, the integral for the enclosed region can be written in the form below.

$A = \displaystyle\int_{−1}^2 [(y − 1) − (y^2 − 3)] dy$

Notice that this integral is written in terms of $y$, and therefore includes the expression $dy$ in the integrand. When solving for bounded areas using functions of $y$, remember that the integrand is equal to the function on the right minus the function on the left. Solving the integral for the area between the functions, we arrive at the answer below.

$\displaystyle\int_{−1}^2 (−y^2 + y + 2) dy$

$\left[−\dfrac{1}{3}y^3 + \dfrac{1}{2}y^2 + 2y \right]_{−1}^2$

$\left(\dfrac{8}{3} + 2 + 4 \right) − \left(\dfrac{1}{3} + \dfrac{1}{2} − 2 \right)$

$A = 4.5$

The correct answer is (D). Notice that there is an intersection point at $x = \frac{π}{4}$ in the interval that we are working with.



This means the integral expression for the area between the curves will need to be written as two separate integrals.

$A = \displaystyle\int_0^{\frac{π}{4}} (\cos(x) − \sin(x))dx$ $+~\displaystyle\int_{\frac{π}{4}}^{\frac{π}{2}} (\sin(x) − \cos(x))dx$

Solving the integral and then applying the first fundamental theorem, we arrive at the answer below.

$[\sin(x) + \cos(x)]_0^{\frac{π}{4}}$ $+~[−\cos(x) − \sin(x)]_{\frac{π}{4}}^{\frac{π}{2}}$

$(\sqrt{2} − 1) + (−1 + \sqrt{2})$

$A = 2\sqrt{2} − 2$