Difficulty Level – 5: Very Difficult
Directions: Solve each problem and then click on the correct answer. You are permitted to use a calculator on this test.
Congratulations - you have completed .
You scored %%SCORE%% out of %%TOTAL%%.
Your performance has been rated as %%RATING%%
Your answers are highlighted below.
Question 1 |
If $a = 3c − 4$ and $b = 2(3 − c)$ what is $3b$ expressed in terms of $a?$
$10 − 2a$ | |
$5 − 2a$ | |
$10 − a$ | |
$\dfrac{10}{3}−\dfrac{2a}{3}$ | |
$\dfrac{5}{3}−\dfrac{5a}{3}$ |
Question 1 Explanation:
The correct answer is (A). Here we are given a system of equations and asked to solve in terms of one of the given variables. It is important to note that we are looking for 3$b$ and not solving for $b$ alone. We will begin by solving for $c$ in terms of $a$ using the first equation. We will then substitute this value into the second equation so as to eliminate the $c$ variable and be left with only a’s and b’s. We will then solve for 3$b$:
$\dfrac{a+4}{3}=c$
$\to b=2 \Biggl( 3-\left(\dfrac{a+4}{3} \right) \Biggl)$
$=2 \left( \dfrac{9}{3}-\dfrac{a+4}{3} \right)$
$=2 \left( \dfrac{5-a}{3} \right)$
Here we will multiply both sides by 3 so we have our target value of 3b on one side, and we will then simplify the expression on the other side:
$3b = 2(5 − a)$
$= 10 − 2a$
$\dfrac{a+4}{3}=c$
$\to b=2 \Biggl( 3-\left(\dfrac{a+4}{3} \right) \Biggl)$
$=2 \left( \dfrac{9}{3}-\dfrac{a+4}{3} \right)$
$=2 \left( \dfrac{5-a}{3} \right)$
Here we will multiply both sides by 3 so we have our target value of 3b on one side, and we will then simplify the expression on the other side:
$3b = 2(5 − a)$
$= 10 − 2a$
Question 2 |
Let the function $f(h, k)$ be defined as $f(h, k) = (h − K^2) + hk$.
For every $x$ and $y$, what does the function $f((x^2 + 2y^2),(x^2 − y^2))$ evaluate to?
$x^4 + x^2y^2 + 7y^4$ | |
$x^4 + 2x^2y^2 + 9y^4$ | |
$2x^4 + 2x^2y^2 − 2y^4$ | |
$x^4 − 2x^2y^2 − 7y^4$ | |
$2x^4 − xy + 9y^4$ |
Question 2 Explanation:
The correct answer is (A). In this case we will use the definition provided to substitute values and evaluate the function:
Set $h = (x²+ 2y² )$ and $k = (x² − y²)$ and make the appropriate substitutions.
$f((x^2 + 2y^2), (x^2 - y^2))$
$= [(x^2+2y^2) - (x^2-y^2)]^2 $ $ + \, [(x^2+2y^2)(x^2-y^2)]$
$= [3y^2]^2 $ $ + \, [x^4 + 2x^2y^2 $ $ - \, x^2y^2-2y^4]$
$= 9y^4 + x^4 + 2x^2y^2 $ $ - \, x^2y^2 - 2y^4$
$= x^4 + x^2y^2 + 7y^4$
Set $h = (x²+ 2y² )$ and $k = (x² − y²)$ and make the appropriate substitutions.
$f((x^2 + 2y^2), (x^2 - y^2))$
$= [(x^2+2y^2) - (x^2-y^2)]^2 $ $ + \, [(x^2+2y^2)(x^2-y^2)]$
$= [3y^2]^2 $ $ + \, [x^4 + 2x^2y^2 $ $ - \, x^2y^2-2y^4]$
$= 9y^4 + x^4 + 2x^2y^2 $ $ - \, x^2y^2 - 2y^4$
$= x^4 + x^2y^2 + 7y^4$
Question 3 |
Let $x \gt 1$
What is the value of:
$\log_x \left(\dfrac{x^4}{x^{10}}\right)$
$− 6$ | |
$− 4$ | |
$\dfrac{1}{2}$ | |
$4$ | |
$6$ |
Question 3 Explanation:
The correct answer is (A).
$\log_x \left(\dfrac{x^4}{x^{10}}\right) = y$, where $y$ is what we are trying to find...
Rewrite in exponential form:
$x^y = \dfrac{x^4}{x^{10}}$
$x^y = \dfrac{1}{x^6}$
$x^y = x^{-6}$
$y = -6$
$\log_x \left(\dfrac{x^4}{x^{10}}\right) = y$, where $y$ is what we are trying to find...
Rewrite in exponential form:
$x^y = \dfrac{x^4}{x^{10}}$
$x^y = \dfrac{1}{x^6}$
$x^y = x^{-6}$
$y = -6$
Question 4 |
If $(a^2 − b^2)^2 = 25$ and $a^2b^2 = 6$, what is the value of $a^4 + b^4?$
$−22$ | |
$−12$ | |
$3$ | |
$25$ | |
$37$ |
Question 4 Explanation:
The correct answer is (E). Begin by foiling the first expression:
$a^4 − 2a^2b^2 + b^4 = 25$
We know that $a^2 b^2 = 6$, so make that substitution as well:
$a^4 −2(6) + b^4 = 25$
$a^4 + b^4 = 37$
$a^4 − 2a^2b^2 + b^4 = 25$
We know that $a^2 b^2 = 6$, so make that substitution as well:
$a^4 −2(6) + b^4 = 25$
$a^4 + b^4 = 37$
Question 5 |
A class contains 2 boys for every 1 girl. 75% of the boys have taken Algebra 2, whereas 50% of the girls have taken Algebra 2. What is the ratio of boys who have taken Algebra 2 to girls who have taken Algebra 2?
$1:1$ | |
$1:2$ | |
$2:1$ | |
$3:2$ | |
$3:1$ |
Question 5 Explanation:
The correct answer is (E). Because we are provided only the ratio of boys to girls, we can provide our own number of boys and girls so long as it satisfies the provided ratio. We will assume there are 10 girls, which means there are 20 boys. Now, ¾ of the boys would be 15 and ½ of the girls would be 5. The ratio of 15:5 is 3:1.
Question 6 |
At summer camp, Ryan made a circular tablecloth for a circular table that is 3 feet in diameter. The circular tablecloth extended beyond the edge of the table by a fixed amount all the way around. The tablecloth was cut from a single piece of square fabric with sides that were 75 inches long. After the tablecloth was removed, 4104 square inches of the original fabric remained.
How far did the circular tablecloth extend beyond the table’s edge when properly centered?
$5 \text{ inches}$ | |
$4 \text{ inches}$ | |
$2 \text{ inches}$ | |
$3 \text{ inches}$ | |
$6 \text{ inches}$ |
Question 6 Explanation:
The correct answer is (B).
$\text{Area of Square} $ $ − \, \text{Area of Tablecloth} $ $ = 4104 \text{ in}^2$
$\text{Area of Tablecloth} $ $ = \text{Area of Square} − 4104$
$\text{Area of Tablecloth } $ $ = 75 \ast 75 − 4104$
$\text{Area of Tablecloth} $ $ = 1521 \text{ in}^2$
The tablecloth is circular. Therefore, we have:
$\pi \ast r^2=1521$
$r = 22.00 \text{ inches}$
The table has a diameter of 3 feet or 36 inches. This means it has a radius of 18 inches.
$\text{Amount of extension} $ $ = 22.00 − 18 = 4 \text{ inches}$
The tablecloth extends 4 inches beyond the table’s edge.
$\text{Area of Square} $ $ − \, \text{Area of Tablecloth} $ $ = 4104 \text{ in}^2$
$\text{Area of Tablecloth} $ $ = \text{Area of Square} − 4104$
$\text{Area of Tablecloth } $ $ = 75 \ast 75 − 4104$
$\text{Area of Tablecloth} $ $ = 1521 \text{ in}^2$
The tablecloth is circular. Therefore, we have:
$\pi \ast r^2=1521$
$r = 22.00 \text{ inches}$
The table has a diameter of 3 feet or 36 inches. This means it has a radius of 18 inches.
$\text{Amount of extension} $ $ = 22.00 − 18 = 4 \text{ inches}$
The tablecloth extends 4 inches beyond the table’s edge.
Question 7 |
A company’s profits increased by 12% from 2010 to 2011 and by 18% from 2011 to 2012. By what percent did the company’s profits increase from 2010 to 2012?
$10\%$ | |
$12\%$ | |
$24\%$ | |
$30\%$ | |
$32\%$ |
Question 7 Explanation:
The correct answer is (E). The most straightforward method for solving problems of this type is to begin by supplying our own initial value prior to any increase or decrease in profits. Because percents are essentially a division by 100, choosing 100 as our starting value for any percent problem is wise. We are told that for the first year, the percent rose by 12%, so:
$100 * 0.12 = 12$
So at the start of 2011, the company now has 112, for which there is another increase of 18%, so:
$112 * 0.18 = 20.16$
Final amount in 2012:
$= 112 + 20.16 = 132.16$
To find the overall percent increase, we find the total increase, then divide this value by the original amount, so:
$132.16 − 100 = 32.16$
$32.16 ÷ 100 = 0.32$
$= 32\%$
$100 * 0.12 = 12$
So at the start of 2011, the company now has 112, for which there is another increase of 18%, so:
$112 * 0.18 = 20.16$
Final amount in 2012:
$= 112 + 20.16 = 132.16$
To find the overall percent increase, we find the total increase, then divide this value by the original amount, so:
$132.16 − 100 = 32.16$
$32.16 ÷ 100 = 0.32$
$= 32\%$
Question 8 |
What is the matrix product of:
$
\begin{bmatrix}
x & 2x \\
−y & y \\
\end{bmatrix}
$ and
$
\begin{bmatrix}
x & x \\
3 & y \\
\end{bmatrix}
$
Recall that when multiplying two 2x2 matrices:
$
\begin{bmatrix}
a & b \\
c & d \\
\end{bmatrix}
\ast
\begin{bmatrix}
w & x \\
y & z \\
\end{bmatrix}
=$
$
\begin{bmatrix}
a*w+b*y & a*x+b*z \\
c*w+d*y & c*x+d*z \\
\end{bmatrix}
$
$
\begin{bmatrix}
x+6 & x+y \\
−xy+3y & xy+y^2 \\
\end{bmatrix}
$ | |
$
\begin{bmatrix}
x^2+6x & x^2+2xy \\
−xy+3y & −xy+y^2 \\
\end{bmatrix}
$ | |
$
\begin{bmatrix}
x^2+6x & −xy+3y \\
x^2+2xy & −xy+y^2 \\
\end{bmatrix}
$ | |
$
\begin{bmatrix}
x^2 & 2x^2 \\
−3y & y^2 \\
\end{bmatrix}
$ | |
$
\begin{bmatrix}
x^2 & −3y \\
2x^2 & y^2 \\
\end{bmatrix}
$ |
Question 8 Explanation:
The correct answer is (B). When asked to find the product of 2 matrices, we must first check to make sure we are capable of doing so. Matrices can be multiplied when the number of columns of the first matrix is equal to the number of rows of the second matrix. Here we have a 2×2 and a 2×2 so the resulting product will be a 2×2 matrix. To calculate the product of two 2×2 matrices let’s first consider the general form:
$ \begin{bmatrix} a & b \\ c & d \\ \end{bmatrix} \ast \begin{bmatrix} w & x \\ y & z \\ \end{bmatrix} =$ $ \begin{bmatrix} a*w+b*y & a*x+b*z \\ c*w+d*y & c*x+d*z \\ \end{bmatrix} $
So, in our case we have:
$ \begin{bmatrix} x*x+2x*3 & x*x+2x*y \\ -y*x+y*3 & -y*x+y*y \\ \end{bmatrix} $
$= \begin{bmatrix} x^2+6x & x^2+2xy \\ -xy+3y & -xy+y^2 \\ \end{bmatrix} $
$ \begin{bmatrix} a & b \\ c & d \\ \end{bmatrix} \ast \begin{bmatrix} w & x \\ y & z \\ \end{bmatrix} =$ $ \begin{bmatrix} a*w+b*y & a*x+b*z \\ c*w+d*y & c*x+d*z \\ \end{bmatrix} $
So, in our case we have:
$ \begin{bmatrix} x*x+2x*3 & x*x+2x*y \\ -y*x+y*3 & -y*x+y*y \\ \end{bmatrix} $
$= \begin{bmatrix} x^2+6x & x^2+2xy \\ -xy+3y & -xy+y^2 \\ \end{bmatrix} $
Question 9 |
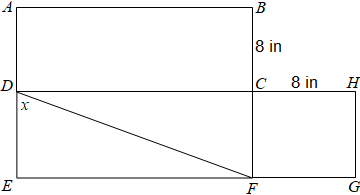
The figure above consists of rectangle $ABCD$, rectangle $CDEF$, and square $CFGH$. Rectangle $ABCD$ has an area of $96 \text{ in}^2.$ In degrees, what is the value of $x \, (∠FDE)$? Round to the nearest whole number.
$24$ | |
$33$ | |
$56$ | |
$61$ | |
$68$ |
Question 9 Explanation:
The correct answer is (C). Using the given area of the top rectangle, we can solve for sides $AB$, $CD$, and $EF$:
$96 = 8 * AB$
$AB = 12$
$CD = AB = 12$ (opposite sides of a rectangle are congruent)
$EF = CD = 12$ (opposite sides of a rectangle are congruent)
$CH = 8$ (given)
$CF = CH = 8$ (all sides of a square are congruent)
$DE = CF = 8$ (opposite sides of a rectangle are congruent)
$\Delta DEF$ is a right triangle (interior angles of a rectangle are $90°$)
$\tan x = \dfrac{\text{opposite}}{\text{adjacent}} = \dfrac{EF}{DE} $ $ = \dfrac{12}{8} = 1.5$
$x = \tan^{-1}(1.5) = 56.3°$
$96 = 8 * AB$
$AB = 12$
$CD = AB = 12$ (opposite sides of a rectangle are congruent)
$EF = CD = 12$ (opposite sides of a rectangle are congruent)
$CH = 8$ (given)
$CF = CH = 8$ (all sides of a square are congruent)
$DE = CF = 8$ (opposite sides of a rectangle are congruent)
$\Delta DEF$ is a right triangle (interior angles of a rectangle are $90°$)
$\tan x = \dfrac{\text{opposite}}{\text{adjacent}} = \dfrac{EF}{DE} $ $ = \dfrac{12}{8} = 1.5$
$x = \tan^{-1}(1.5) = 56.3°$
Question 10 |
The function $f(x) = \cos²(x) * \csc (x)$ can be rewritten as which of the following?
$\sin (x) * \cos (x)$ | |
$\cos (x) * \cot (x)$ | |
$\cot (x) * \tan (x)$ | |
$\sin (x) * \cot (x)$ | |
$\sec (x) * \csc (x)$ |
Question 10 Explanation:
The correct answer is (B).
$f(x) = \cos^2(x) \ast \csc(x)$
$f(x) = \cos^2(x) \ast \dfrac{1}{\sin(x)}$
$f(x) = \cos(x) \ast \dfrac{\cos(x)}{\sin(x)}$
$f(x) = \cos(x) \ast \cot(x)$
Note: If you struggle with this sort of problem, you could also choose an arbitrary value for $x$ (e.g. $x$ = 33°). Plug it into the original equation and find the result. Then plug the same $x$ value into each answer choice until you get a matching result.
$f(x) = \cos^2(x) \ast \csc(x)$
$f(x) = \cos^2(x) \ast \dfrac{1}{\sin(x)}$
$f(x) = \cos(x) \ast \dfrac{\cos(x)}{\sin(x)}$
$f(x) = \cos(x) \ast \cot(x)$
Note: If you struggle with this sort of problem, you could also choose an arbitrary value for $x$ (e.g. $x$ = 33°). Plug it into the original equation and find the result. Then plug the same $x$ value into each answer choice until you get a matching result.
Once you are finished, click the button below. Any items you have not completed will be marked incorrect.
There are 10 questions to complete.
|
List |
Next Practice Test:
ACT Math Practice Test 10 >>
More Practice Tests:
ACT Math – Main Menu >>
ACT Science Practice >>
ACT English Practice >>
ACT Reading Practice >>
